William Herschel’s discovery of the planet Uranus in 1781 strengthened a rule of thumb proposed shortly before, called the Titius-Bode law, which was intended to determine the distance of the planets from the Sun—even if the law had no scientific basis other than extrapolation of the distances to the Sun of the known planets. Titius-Bode’s law stated that, if there was a planet beyond Saturn, the mean radius of its orbit should measure 2.94 billion kilometres, more or less; and it turned out that this was a good approximation for the distance of Uranus from the Sun. But the same law also predicted the existence of another planet between Mars and Jupiter; the orbits of these planets are too far apart – almost 550 million kilometres – and there was room for another planet. Specifically, the Titius-Bode law stated that such a planet could be approximately 420 million kilometres away from the Sun. In 1796, at an astronomical congress, a recommendation was made to search for it, and many of the astronomical observatories of the time pointed their telescopes at the marked orbit in an attempt to find it. But searching for a planet with only its supposed distance from the Sun is like looking for a needle in a haystack, and years went by without success for the hard-working “celestial policemen”—a nickname they liked to use.
Fortune changed on 1 January 1801, a date with a whiff of something very much to the liking of millenarians and astrologers. On that day, Giuseppe Piazzi, from the modest observatory in Palermo, spotted between Mars and Jupiter what at first seemed to him to be a comet, but which later turned out to be something else. Piazzi observed it for several more nights, but the object moved closer to the Sun and was no longer visible.
Many thought that the object Piazzi found would be the planet whose existence was predicted by the Titius-Bode law, and in fact Piazzi was ready to name it, choosing a first and last name. He chose the name Ceres, goddess of seeds, a Roman version of the Greek Demeter who, as Sicily was one of Rome’s granaries, had been very popular there; and Ferdinandea, after Ferdinand IV of Naples and III of Sicily, king of the two Sicilies, son of Charles III of Spain and Piazzi’s patron surname. The new member of the solar system soon lost the surname and is known today as Ceres, simply. But there was a much bigger problem with Ceres than the name. The data that Piazzi had been able to collect were very sparse and made it extremely difficult to calculate its orbit; nevertheless, the astronomers did the calculations. It was estimated that the object would be visible again that year in early September, but by that time Ceres was nowhere to be seen.
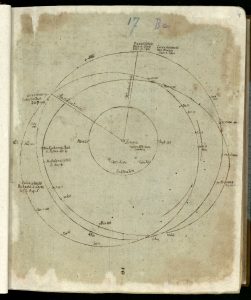
Then Carl Friedrich Gauss, then a mathematician but not an astronomer, stepped in. It is possible that he became aware of the problem that September. By October he had developed a method for calculating the orbit of a celestial body moving around the sun using a few known observations. Gauss sent his calculations to Franz von Zach, director of the Astronomical Observatory in Gotha; on 7 December 1801 he located Ceres right where Gauss’s mathematics had predicted it would be, although due to bad weather he was unable to verify the finding until 31 December 1801. After Ceres was rediscovered thanks to Gauss’s calculations, it was found to be too small –it is just over 900 kilometres in diameter, compared to the moon’s nearly 3,500 kilometres– so small that astronomers found it inappropriate to call it a planet. Herschel proposed calling it an “asteroid”, which somewhat annoyed Piazzi who preferred the appellation “planetoid”. Other asteroids were soon discovered – Piazzi’s term did not prosper—and the well-stocked classical pantheon provided names for all of them: Pallas, Juno, Vesta… Gauss was in charge of calculating the orbits of the first ones that were discovered and, for that reason, he was given the honour of naming one of them: Vesta.
Gauss published his method eight years later, when he was already Director of the Göttingen Astronomical Observatory, under the title The theory of the motion of celestial bodies moving around the Sun in conic sections. The method, called least squares, allows the orbit of the celestial body to be determined from only three or four good observations and is still used today to determine the orbits of artificial satellites.
Gauss’s now famous perfectionism – one of whose mottos was “Pauca et matura”: “little but well done” – caused him to delay the publication of the method by eight years. During this time he refined it and brought it to a degree of perfection very much to his liking. In the preface Gauss justified the delay: “In the hope that a further study of this method would bring its solution to a greater degree of generalisation, simplicity and elegance”. However, the mathematical perfection that Gauss finally achieved is not to everyone’s liking: “Gauss’s computational triumphs,” wrote Ivars Peterson in Newton’s Clock, “brought him immediate and lasting recognition as Europe’s greatest mathematician and a comfortable position as professor of astronomy and director of the Göttingen Observatory, where he lived modestly for the rest of his long and productive life. In no hurry to see his ideas published, whether in mathematics, astronomy or physics, Gauss relentlessly reworked his results again and again until they were polished to perfection. Clarifying his thoughts step by step and eliminating all but the essential elements, he erased all traces of the path he had followed to arrive at his discoveries. No scaffolding ever spoiled the elegant structures he had so patiently built. This austere style, which now remains in mathematics, may be one of Gauss’s less happy legacies. To the uninitiated, the rigid abstraction involved in much contemporary mathematics is virtually impenetrable. Only a select few dare to confront its dense and tightly woven fabric”.
The Disquisitiones Arithmeticae that Gauss had also published in 1801 and the astronomical calculations that allowed him to locate Ceres are a magnificent example to illustrate the way in which Gauss understood mathematics; it is a science with two sides: on the one hand it is a game of the spirit, on the other, an irrationally effective and indispensable tool to study and understand nature.
References
A.J. Durán, Pasiones, piojos, dioses… y matemáticas, Destino, Barcelona, 2009.
I. Peterson, El reloj de Newton. Caos en el sistema solar, Alianza, Madrid, 1995.

Leave a Reply