In 2022, a little more than five years after writing this entry, Maryna won the Fields Medal, with the Committee highlighting precisely this work that we have discussed. I am convinced of the value of her work. If someone had posed me the problem of finding this function, I would have pointed to Noam Elkies as the best researcher to find it. But Elkies did not know how to do it. Maryna’s merit is indisputable and the fact that her work is after all so transparent only adds to my admiration.
I sometimes tell my students that if I were their age I would start studying modular forms right away. They are the great engine of mathematics today. Where the most mysterious questions reside. They permeate everything. I don’t think many of them have paid any attention to me, there is not a single course on modular forms in Seville, and I have managed to devote two or three classes to them at most one year.
Kepler problem
A few months ago Maryna Viazovska surprised everyone with the solution of the sphere packing problem in dimension 8, a few weeks later, using her methods, she and four other collaborators Harvey Cohn, Abhinav Kumar, Stephen D. Miller and Danylo Radchenko solved the 24-dimensional case.
The problem goes back to Kepler who in 1611 wondered how best to pack spheres in ordinary space.
In general in dimension \(d\), given a discrete set of points \(X\subset\mathbf{R}^d\) such that the distance \(d(x,y)\) between two distinct points of \(X\) is always \(\ge2\), we consider the set \(P=\bigcup_{x\in X}B(x,1)\) formed by the balls with centre at the points of \(X\) and radius \(1\). These balls are disjoint and \(P\) is said to be a ball-packing. Its density \(\Delta_P\) is defined as the upper limit of the quotients of the measures covered by the balls
$$\Delta_P:=\limsup_{r\to+\infty}
\frac{\mathop{\rm Vol}(P\cap B(0,r))}{\mathop{\rm Vol}(B(0,r))}$$
That is, \(\Delta_P\) measures the fraction of the space occupied by the balls.
Our problem is to calculate \(\Delta_d\), that is, the supremum of these densities \(\Delta_d=\sup_P \Delta_P\) when the balls are in dimension \(d\). It is known that this supreme density is reached, i.e. it is a maximum.
Progress on the Kepler problem
Dimension 1, \(\Delta_1=1\).
In dimension 1 the problem is trivial. The balls are segments and can be arranged to occupy the whole space \(\mathbf{R}\).
Dimension 2, \(\Delta_2=\frac{\pi}{\sqrt{12}}\).
In this case it has been suspected since antiquity that the best density is given by the familiar hexagonal arrangement in which each disc touches six other discs.
However, the first proof that \(\Delta_2=\pi/\sqrt{12}\) was not given until 1910 by A. Thue. Some experts considered it incomplete and the first rigorous proof was given by L. Fejes Tóth in 1943.
The difficulty is to prove that no other arrangement of discs can give a higher density.
Dimension 3, \(\Delta_3=\frac{\pi}{\sqrt{18}}\).
In dimension 3 the problem is much more complicated. In 1611 Johannes Kepler in his work On the Hexagonal Snowflake conjectured that the best way to pack spheres was the usual way in greengrocers’ shops to display oranges, with a density of \(\frac{\pi}{\sqrt{18}}\). This Kepler conjecture has had a very interesting history. The proof was published by T. Hales in 1998.
T. Hales sent his proof to the journal Annals of Mathematics. The proof was unusual. Recall that it is a proof that no other configuration than the conjectured one gives a higher density. It was a proof by cases that took about 250 pages, apart from the codes needed to check certain inequalities by computer. Annals selected as referees a group of 12 mathematicians. After four years, this team led by Fejes Tóth announced that they had found no errors in the proof, although they could not completely guarantee that there were none, especially in the codes.
Finally Annals published the proof. The story does not end here. In 2015 T. Hales together with 21 co-authors have published a full formal proof of Kepler’s conjecture. This can be checked by automatic proof software.
Dimensions \(d=1\), \(d=2\) and \(d=3\) were the only known exact values before Maryna’s work. Maryna has proved that \(\Delta_8=\pi^4/384\) and with her collaborators has also proved \(\Delta_{24}=\pi^{12}/12!=\pi^{12}/479001600\).
Results prior to Maryna’s work
Maryna’s work rests on two cornerstones.
First: in certain dimensions a particularly good packing is available. This provides lower bounds for \(\Delta_d\), lower because we do not know for certain that they give the optimal packing. These packings correspond to \(\Lambda\) lattices. This means that the centres of the balls are located in the linear combinations with integer coefficients of a set of basis vectors.
Second: Reasonings of H. Cohen and N. Elkies provide an upper bound.
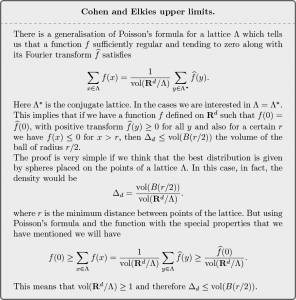
After proving this principle, H. Cohn and N. Elkies search for suitable functions that give better than the known bounds. In the figure we see the graph of their upper bounds versus the best lower bounds obtained from particular packings.
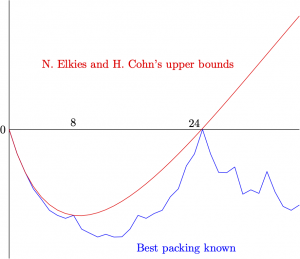
Elkies and Cohn saw that, as we can see in the figure, their bounds were very close to the lower bound in the case of dimensions 8 and 24. The equality in the above reasoning would imply that in the above Poisson sums all the terms except the one corresponding to the origin are \(=0\) for \(f\) and for \(\widehat{f}\).
This made them conjecture that there would be some magic functions that would give exact equality with the best known bundles, which were associated to two lattices very famous for their symmetry properties. So much so that although they could not find these magic functions, they were so confident of finding them in the future that they entitled their paper New upper bounds on sphere packings. I. But they were unable to publish the sequel II.
Maryna and modular forms
Modular forms are holomorphic functions defined on the two-sided upper half-plane.
- They have infinite symmetries associated with non-Euclidean geometry when considered as functions of the variable \(\tau\).
- They have a power series development \(\sum a_nq^n\) in the variable \(q=e^{2\pi i\tau}\) whose coefficients \(a_n\) contain very interesting arithmetic properties.
If you want to learn more, you can start by watching the videos of Don Zagier’s lectures that I include at the end of this post. Don Zagier is Maryna’s thesis supervisor. We already know where he found the necessary tools to find the magic function.
Modular forms are very closely associated with lattices, which might lead one to suspect their relation to the magic functions of Cohn and Elkies.
However, it was not easy to find them, just think that Elkies must have tried (anyone who doesn’t know him can look up his interventions on MathOverflow to be amazed every day). This makes Maryna’s work all the more remarkable.
Maryna Viazovska was born in Ukraine in 1984. She studied in Kiev and received her doctorate in Bonn under the direction of Don Zagier (Modular forms and Special Cycles). For this work she received the Salem Prize, but she also has important previous work on spherical designs.
Learn more
[1] Maryna’s paper has an excellent introduction which we have used in our presentation:
M. Viazovska, The sphere packing problem in dimension 8. Ann. of Math. 185 (2017), 991–1015.
You can also find it in arXiv.
[2] We have also widely used the (somewhat more explicit than our own) dissemination article in the AMS Notices:
H. Cohn, A Conceptual Breakthrough in Sphere Packing, Notices Amer. Math. Soc. 64 (1917), 102–115.
[3] Another interesting article on the subject with interviews with H. Cohn, Abhinav Kumar, Stephen D. Miller and Maryna Viazovska appeared in the Dutch journal Nieuw Archief voor Wiskunde.
[4] For those who want more on modular forms I recommend Don Zagier’s four lectures on the subject at the International Centre for Theoretical Physics: The Magic of Modular Forms.
[4a] Classical Modular forms and some of their applications in arithmetic.
[4b] Modular forms, differential equations and the irrationality of zeta.
[4c] Quasimodular forms and counting problems in topology and group theory.
[4d] Mock modular forms and the string theory of black holes.
Some lighter stuff:
[5] A clip on Youtube to understand spheres in higher dimensions.
[6] A post on the blog Gaussianos.
[7] A article on Quanta magazine.



Leave a Reply