And waves became particles

In a previous post we described how Einstein, in order to explain various phenomena including the photoelectric effect, recovered Newton’s idea that light is composed of small particles (today called photons) to which he assigned an energy proportional to the frequency \(\omega\) of light, \(E=h\omega\) where \(h\) is Planck’s constant.

Einstein himself entitled the paper “On a Heuristic Viewpoint Concerning the Emission and Transformation of Light“, emphasising the word heuristic.  In fact, in an undated letter, but probably between 18 and 25 May 1905, (see letter 27 by clicking here) Einstein wrote to his friend Conrad Habicht

[…] In exchange I promise you four articles, the first of which I will send you soon, as I will soon receive the complimentary reprints. The article deals with radiation and the energetic properties of light and is very revolutionary.

And it was indeed very revolutionary (or, if you like, very against the tide) because after the work of Young and Fresnel it was assumed by all physicists that light was a wave, something that strengthened James Clerk Maxwell’s electrodynamic theory. n particular, Young’s double-slit experiment proved beyond doubt the wave property. This experiment consisted of launching a wave (light) that impacted on a wall with two small slits. On reaching the two slits (whose opening is small enough) two waves are generated which interfere with each other and which, on impacting on a second screen, generate the famous interference pattern. If we were to repeat the experiment with classical particles (e.g. marbles or balls) what we would see are two maxima corresponding to the particles that have passed through each slot.

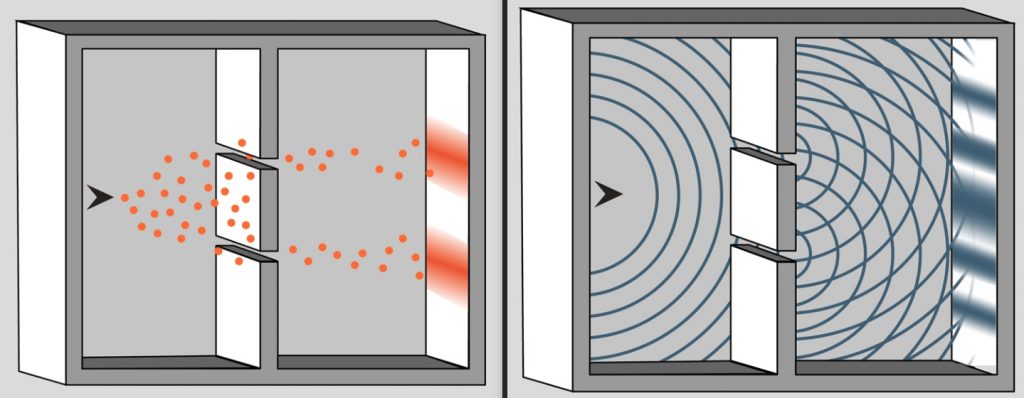
The simplest way to explain the interference phenomenon is to add the waves together. Thus, if the waves arrive at the screen in phase, then at the points where both have their peak (the maximum value of the amplitude) a wave with a greater amplitude is obtained and therefore there is a greater intensity of the light. Conversely, at the points where they arrive in counter-phase (i.e. out of phase by 90 degrees or \(\pi/2\) radians), both waves annihilate and we see a shadow.

In his “revolutionary” work, Einstein proposed that, under certain conditions, light had to be considered as composed of small particles and not as a wave, and this was something that physicists were not willing to accept easily. Robert Milikan himself, who tested the Einsteinian theory of the photoelectric effect, wrote in 1949: “I spent ten years of my life testing Einstein’s 1905 equation, and contrary to all my expectations I was compelled, in 1915, to proclaim the undoubted experimental verification, however unreasonable it was, since it seemed to violate everything we knew about the interference of light”. Similarly, other well-known physicists of the time, including Max Planck, Arnold Sommerfeld, Joseph J. Thomson, Hendrik Lorentz, to name but a few of the most influential, did not like Einstein’s corpuscular idea. But the quanta came to stay. And they did.

In 1909 Einstein wrote two articles on the wave-particle duality of electromagnetic radiation: In the first, entitled “Current state of the radiation problem“ (publicado en el número 10 de Physikalische Zeitschrift) (published in issue 10 of Physikalische Zeitschrift), Einstein studies, on the basis of Planck’s Law, Boltzmann’s Theory and thermodynamics, two closely related problems:

1. the fluctuation of energy inside a cavity (black body) with radiation
2. the motion of a reflecting plate moving inside a cavity.

where \(\rho\) is the energy density and \(f\) is its surface. From this expression Einstein explained that although the second term (which corresponds to the zone of validity of the classical Rayleigh formula that we have already talked about in this other post) can be easily explained using Maxwell’s electromagnetic theory, the first one (corresponding to the zone of validity of Wien’s formula) cannot. Moreover, if only the first term \(h\rho\omega\) were to appear, this would be a consequence of considering radiation as constituted by corpuscles with energy \(h\omega\). Now, as Einstein himself explained, the first term cannot be ignored, because for values of \(\omega=6\cdot 10^{14}\) Hz and a temperature of \(T=1700\) Kelvin degrees, it is \(6.5\cdot 10^{7}\) times bigger than the second one, that is to say, the corpuscular hypothesis took on a lot of weight. In other words, light apparently behaved as if it were both a wave and a particle.

How to resolve this apparent contradiction? Einstein himself was aware of this problem, as he wrote to Lorentz on 30 March 1909, sending him a copy of the above-mentioned paper:

Enclosed with this letter I am sending you a short article on radiation theory, which is the insignificant result of years of meditation. I have not been able to work my way to a true understanding of the subject. But I am sending it to you anyway, and even ask you to have a quick look at it, for the following reason: The article contains several arguments from which it seems to me to follow that not only molecular mechanics, but also Maxwell-Lorenz electrodynamics, cannot agree with the radiation formula.

Indeed, the main problem of Einstein’s theory of light quanta was related to the theoretical deduction of Planck’s formula: there was still no way to avoid the use of electromagnetism, a theory with which Planck’s own formula was in contradiction. In Einstein’s own words at the First Solvay Congress in 1911 [To better understand Einstein’s criticism, we recommend the reader to read the post where Planck’s demonstration of the black body radiation formula is discussed]:

It seems a bit shocking to apply the Boltzmann equation as Planck wants, introducing a probability \(W\) without giving a physical definition of it. If one does so, the Boltzmann equation has no physical meaning. The fact that \(W\) is taken as the number of configurations does not change any of this, because it is not explained how two configurations will be recognised as equally probable.

Actually, the problem of the deduction of Planck’s formula was a recurrent problem in physics at the beginning of the 20th century, and Einstein was no stranger to it. As he himself wrote in his autobiographical notes, “All my attempts to adapt the theoretical foundation of physics to this knowledge failed utterly. It was as if the ground had been removed from under one’s feet, with no solid ground in sight on which to build.”

However, as we have already mentioned, light quanta were here to stay. Experimental confirmation of this fact came about thanks to the research of the American physicist Arthur H. Compton, who published a paper in 1923 in which he explained the results of his work on the diffusion of X-rays (electromagnetic waves like light, but with a very high frequency), which we know today as the Compton effect and which won him the Nobel Prize for Physics in 1927. The effect was as follows: if we irradiated different materials with X-rays (such as graphite, which was the one used by Compton), we observed a change in the wavelength (or frequency) of the X-rays as a function of the scattering angle (see diagram), something inexplicable using the wave theory of light. In the diagram below we see a photon with frequency \(\omega\) colliding with an electron and then deflecting and changing its frequency by \(\omega’\) depending on the scattering angle.

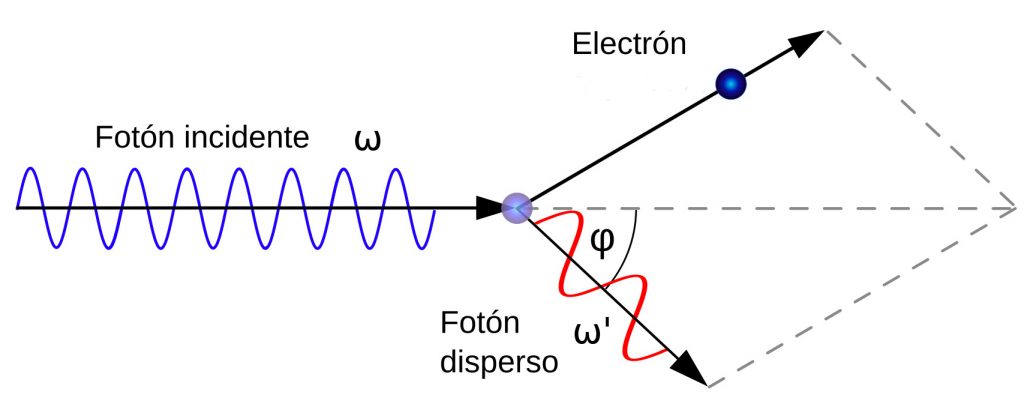
To explain this, Compton took Einstein’s idea and considered X-rays as balls (quanta) of radiation with zero mass but with energy and linear momentum \(h\omega\) and \(h\omega/c\), respectively, which interacted elastically (i.e. energy and momentum were conserved). After some simple mathematics (using the laws of conservation of energy and linear momentum) he obtained results that agreed with his experiments.

This was the definitive experimental confirmation that light was made up of particles. Compton himself presented his results at a meeting of the American Physical Society in December 1923. There, in a lecture entitled “X-ray scattering”, he stated:

Undoubtedly the most important result of this work is, however, the information it provides with respect to electromagnetic radiation. We find that the wavelength and intensity of the scattered rays are what they should be if a quantum of radiation bounced off an electron, just as one billiard ball bounces off another. Not only this, but we actually observe the billiard ball, or electron from which the quantum has bounced back, and find that it moves with exactly the velocity it should if a quantum had hit it. The obvious conclusion would be that X-rays, and so also light, are made up of discrete units, moving in definite directions, each possessing the energy \(h\nu\) and the corresponding momentum \(h\nu/c\). In a recent letter Sommerfeld has expressed to me the opinion that this discovery of the variation of the wavelength of radiation due to diffusion, signifies the death knell of the wave theory of radiation.

But what about the theoretical approach, how to reconcile the two points of view? And Planck’s formula, what to do with it? An outsider to the world of Physics at the beginning of the 20th century would have to come along to solve this last problem. But we will tell you about that in a next post.

Learn more:

José Manuel Sánchez Ron, Historia de la física cuántica: I. El período fundacional (1860-1926), Drakontos, 2001.

J. Mehra y H. Rechenberg, The Historical Development of Quantum Theory, Vol 1. The Quantum Theory of Planck, Einstein, Bohr and Sommerfeld: Its Foundation and the Rise of Its Difficulties 1900-1925, 1982 Springer-Verlag New York Inc.