Travelling through time (and III)

Wormholes

A wormhole is a special class of solutions to the Einstein equations characterised by providing “shortcuts” in space-time. These shortcuts allow, at least theoretically, to pass from one region of space-time to another by unsuspected paths, not necessarily coincident with those marked by geodesics.

The term “wormhole” comes from the American physicist John Wheeler. It is based on interpreting the universe as the skin of an apple and comparing the time it would take for a worm to travel from one point to another at its antipode by moving over the surface or digging an inner hole. The concept has been taken to the cinema on many occasions. The 2014 film Interstellar, directed by Christopher Nolan and starring among others Matthew McConaughey and Anne Hathaway, deserves special attention.

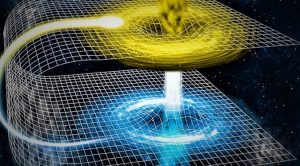
Reader: if you want to fully understand this figure, I suggest you read the explanations given at the end of the post.

 

In 1935, a first wormhole, now called the Einstein-Rosen bridge, was discovered; see [2]. Some time later, in 1962, John Wheeler and Robert Fuller proved that this solution is unstable and would forcibly disintegrate instantaneously as soon as it formed. In 1988, Kip Thorne and his student Michael Morris discovered the hole that bears their names; see [3]. In this case, the solution is “traversable”, i.e. it allows to travel not only from one part of the universe to another, but even from one universe, determined by a particular metric, to another. The way it is usually represented is well known; see Fig. 1.

To date, there is no trace of wormholes in real space-time. However, the General Theory of Relativity says that a rotating black hole can give rise to a wormhole. On the other hand, according to a recent contribution by scientists from the Universities of Buffalo and Yangzhou, there must be “simple” detection methods: since a wormhole connects regions of space-time that are in principle distant, high-mass objects on one side must exert relevant gravitational influence on those on the other side, see [1].

In real space-time there must be enough “nooks and crannies” and “gaps” to suspect the existence of ”alternative” paths not only in spatial directions but also in time. Just as we observe irregularities on the surface of any everyday object, no matter how smooth its outline may appear to be, so we can expect tiny cracks and wrinkles in the temporal dimension. These cracks will eventually produce shortcuts and give rise to microscopic wormholes. Unfortunately, we are talking about tunnels of such a small scale that it is unthinkable to take advantage of their existence for human time travel.

Let’s talk about time travel again

According to Hawking, a wormhole should in theory allow time travel. The possibility arises if one end (denoted \(A\)) has relatively high velocity relative to the other (denoted \(B\)). Thus, the relativistic effect leads to a “mouth” that ages slower than the other for an outside observer. But, for an observer in the interior, the extremes remain synchronised; in particular, an individual travelling from \(A\) to \(B\) “exits” the hole at an earlier time than he or she entered.

Unfortunately, there are many reasons to suspect that this is pure theory (and even speculation). Thus, we are a long way from having the technology to “build” a wormhole; we would therefore have to resort to “natural” wormholes (if any). On the other hand, it seems very difficult to imagine a vehicle capable of safely travelling inside a wormhole under enormous gravitational effects. Another unrealistic aspect is the need for a “return journey”, once the wormhole has transported us (possibly) to a distant part of the universe.

Furthermore, travelling backwards in time is counter-intuitive and even contrary to the laws of physics. It leads to paradoxes. One of the best known is the grandfather paradox: a time traveller goes back into his own past and accidentally kills one of his parents or one of his grandparents, so that he is never conceived and, consequently, is not alive.

For all these reasons, the possibility of retrograde time travel is widely rejected today. In any case, it seems neither probable nor feasible with our current level of scientific and technological knowledge.

Thorne

Kip Stephen Thorne (b. 1940) is an American theoretical physicist, winner of the Nobel Prize in Physics and the Princess of Asturias Award for Technical and Scientific Research. He is considered one of the world’s leading experts on applications of the General Theory of Relativity to astrophysics. He is known for his numerous contributions, for having trained a large number of scientists, and also for his great ability to disseminate ideas and results, including at the level of popular science.

In fact, he was a scientific advisor for the film Interstellar. He later stated that, in order to represent wormholes and the black hole, after talking to the director and the producers, he started to work on the equations that allowed the tracking of light rays, with the intention of not contradicting Einstein’s equations.

In 1975, Thorne was involved in a bet with Hawking in which he defended the existence of black holes in the real Universe. At stake was a subscription to Penthouse magazine. In a 2017 interview, he said that when the theory began to be confirmed by scientific evidence, successive issues began to arrive punctually at home.

Again, some explanations for demanding readers

The topological definition of a wormhole is not intuitive. A wormhole is said to exist in a compact region of space-time when its boundary set is topologically trivial, but its interior is not simply connected (something like a circle without a centre).

Recall that, in the General Theory of Relativity, space-time is determined by Einstein’s equation

$$
R_{\mu\nu} – {1\over2} R g_{\mu\nu} = \kappa T_{\mu\nu} ,
$$
where \(R = g^{\mu,\nu} R_{\mu,\nu}\) is the scalar curvature and \(R_{\mu,\nu}\), \(g_{\mu,\nu}\) and \(T_{\mu,\nu}\) are, respectively, the components of the Ricci tensor, the metric tensor and the energy-momentum tensor.

In geometrical-physical terms, the Einstein-Rosen bridge can be described as follows. Let us first consider the metric of the Schwarzschild black hole
$$
g = -c^2 \left(1-\frac{2GM}{c^2 r} \right) \mathrm{d}t \otimes \mathrm{d}t + \left(1-\frac{2GM}{c^2 r}\right)^{-1}\mathrm{d}r\otimes \mathrm{d}r + r^2 \left(\mathrm{d}\theta\otimes \mathrm{d}\theta + \sin^2\theta\ \mathrm{d}\phi\otimes \mathrm{d}\phi \right) ,
$$
Let us set \(m := GM/c^2\) and let us introduce the new variables \(\tau = ct\) and \(u^2 = r – 2m\). Then \(g\) can be rewritten in the form
$$
g = – \frac{u^2}{u^2 + 2m} \, \mathrm{d}\tau \otimes \mathrm{d}\tau + 4(u^2 + 2m)\,\mathrm{d}u\otimes\mathrm{d}u
+ (u^2 + 2m)^2\left(\mathrm{d}\theta\otimes \mathrm{d}\theta + \sin^2\theta\ \mathrm{d}\phi\otimes \mathrm{d}\phi \right)
$$

and interpreted as a metric specific to a wormhole. Thus, in the \(4\)-dimensional space-time described by the variables \(\tau\), \(u\), \(\theta\) and \(\phi\), we find a hyperplane \(r = 2m\) which divides the regions \(u > 0\) and \(u < 0\) and thus plays the role of a “bridge”, see [1] for more details.

On the other hand, the Morris-Thorne wormhole metric is given in spherical coordinates by the following equality, where \(k\) is a constant:
$$
g = – c^2 \mathrm{d}t \otimes \mathrm{d}t + \mathrm{d}r\otimes \mathrm{d}r + (k^2 + r^2)
\left(\mathrm{d}\theta\otimes \mathrm{d}\theta + \sin^2\theta\ \mathrm{d}\phi\otimes \mathrm{d}\phi \right) .
$$

It is possible to represent (for example) the sections \(\theta = \pi/2\), \(t = \hbox{Const.}\), using an immersion technique. The idea is as follows:

• In polar coordinatese \((\rho,\phi)\), a surface of revolution \(z = z(\rho)\) in \({\bf R}^3\) has the associated metrics $$g_z = \left( 1 + |z_\rho|^2 \right) \mathrm{d}\rho\otimes\mathrm{d}\rho + \rho^2 \mathrm{d}\phi\otimes \mathrm{d}\phi .$$

• For \(\theta = \pi/2\), \(t = \hbox{Const.}\), the Morris-Thorne metrics with \(\rho^2 = k^2 + r^2\) is reduced to $$g_{\rm MT} = \left( 1 – k^2/\rho^2 \right)^{-1} \mathrm{d}\rho\otimes\mathrm{d}\rho + \rho^2 \mathrm{d}\phi\otimes \mathrm{d}\phi .$$

Comparing both expressions and imposing \(z(k) = 0\), we get the function
$$z(\rho) = \pm k \log \left( \frac{1}{k}\left(\rho + \sqrt{ \rho^2 – k^2 }\right) \right), \ \ \rho \geq k,$$

that has been visualised in Fig. 1.

Learn more

1. D.-Ch. Dai, D. Stojkovic, Observing a wormhole, Phys. Rev. D 100, 083513, Published 10 October 2019.
2. A. Einstein, N. Rosen, The particle problem in the General Theory of Relativity, Physical Review 48, 73 (1935).
3. S.W. Hawkings, How to Build a Time Machine, Daily Mail Online, April 2010.
4. M.S. Morris, K.S. Thorne, Wormholes in spacetime and their use for interstellar travel: a tool for teaching general relativity, Amer. J. of Physics 56, 395 (1988).
5. T. Müller, Visual appearance of a Morris-Thorne-Wormhole, August 2004, American Journal of Physics 72 (8).
6. R.H. Price, General relativity primer, American Journal of Physics, 50: 300-329.